The method of calculating the sound field with a numerical solution includes the following stages:

• The pressure at each of the blocked ear-canals is calculated due to a single source at a time. The position of the source is equivalent to the position of a real source.

• A matrix of the ‘electro-acoustic’ response is obtained (2x2 for two speakers and two ears, and 4x4 for four speakers and four ears). e.g. C12 is the response at ear no. 1 due to source no. 2.

• A desired signal is determined. Generally “0“ pressure is assigned to one of the ears, and “1“ to the other ear, i.e. d=[1 0]^T for a 2x2 system, where d is the vector of the desired signals.

• Each source is filtered (via appropriately designed inverse filters) to produce the desired signals with a table of complex pressure values at each frequency. The regularisation value was chosen for each head and loudspeaker arrangement as described below. In all cases the regularisation parameter was chosen to be 0.001 to remove ill conditioning due to symmetric acoustic paths.

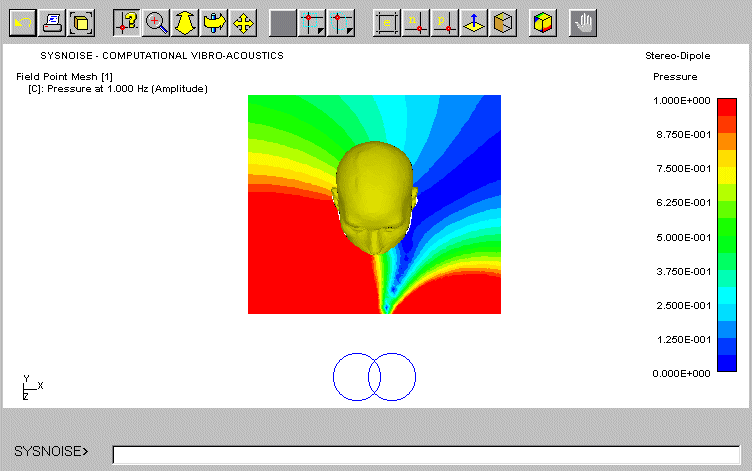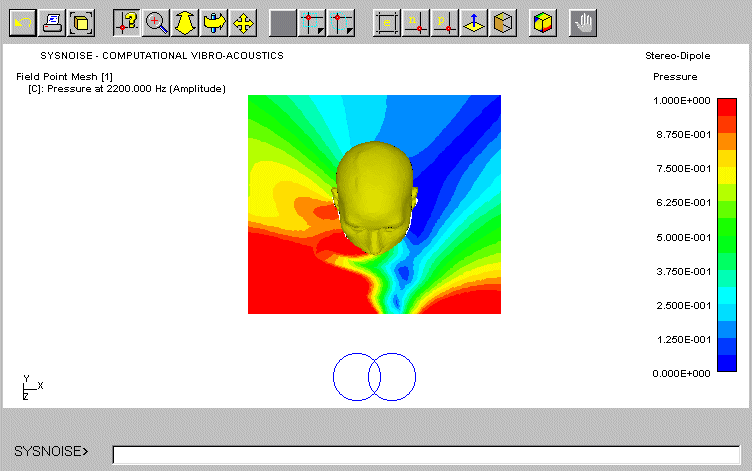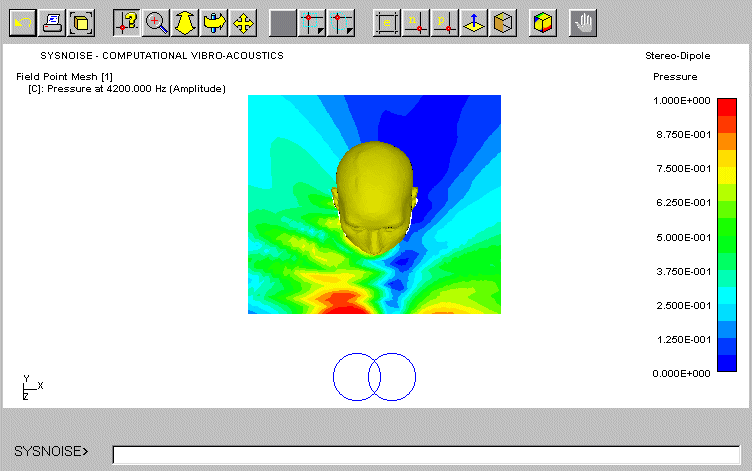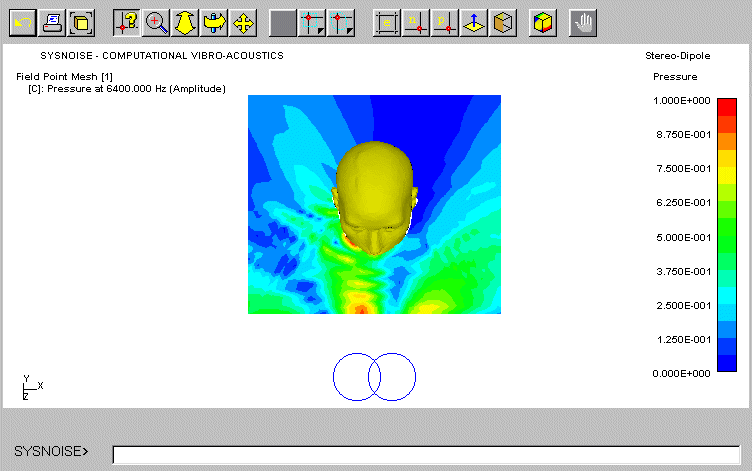
Our goal is to control locally the pressure at the entrance to the ear canal only. Our cross-talk cancellation matrix is designed to produce zero pressure at the right ear of KEMAR and pressure of unity in its left ear. It is seen that the proximity of the speaker divides the pressure zones to the right and left sides of the head. As frequency increases the equalisation zone on the head is reduced, and only accurate attenuation is obtained at the entrance to the ear canal and within the concha. The scale is linear where red is limited to unity and blue to zero pressure. Note that this simulation is ideal, and it does not highlight the problem of loudspeaker behaviour due to the ill-conditioning at low frequencies.
The animation shows the resulting sound field around the head in the frequency domain for the same filtered signals as used in the previous figure. The characteristic common to all frequencies is the symmetry with respect to the centre axis between the sources, i.e. the separation between the right and left channels is preserved even if the head is not positioned in the exact 'sweet-spot' but along this axis.