Introduction

Waves

What is a wave ?

A wave is a disturbance or variation which travels through a medium (air, water or whatever carries the wave). Each little bit of the medium experiences some local oscillations as the wave passes and forward the disturbance to its neighbours. However, each parcel in the medium does not travel with the wave: waves transfer energy without transferring matter.

Examples

Let consider air particles set in motion by a piston. We can see that the particles move back and forth about their equilibrium position, thus creating alternating zones of compression and rarefaction. However, it is the disturbance which travels, not the individual particles. In air, the local oscillations always move in the same direction as the wave. These waves are classified as longitudinal waves, an example of which is given by acoustic or sound waves.

Unlike acoustic waves, radio waves or guitar-string vibrations are transverse waves; that is, the local oscillations are always up and down while waves travel from left to right.

We can see from the above animations that the shape of the waves repeats itself at regular distances. The distance between similar points on adjacent waves is called the wavelength λ. The speed at which the wave goes from one place to the other is called the wave velocity v.

Let's now take a look from the below animations at the red point that bobs up and down with the crests and troughs as the wave passes. The rapidity with which the point moves up and down depends on the wave velocity, but also on the crest-to-crest distance, the wavelength. A measure of how often the oscillating motion repeats at a single place is called the frequency of oscillation, f. Wave velocity, frequency and wavelength are related by

v = f λ

This relation holds for any kind of wave phenomena.

     Two acoustic longitudinal waves with the same wave velocity and two different frequencies.

  Two acoustic longitudinal waves with two different wave velocities and the same frequency.

Sound is a mechanical wave

Sound is due to small pressure variations of the atmosphere. Sound waves are longitudinal waves. They propagate in air at room temperature at about 340 meters per second, that is v = 340 m/s. The range of frequencies audible to human ears lies between about 20 and 20,000 cycles per second (20 Hz to 20 kHz). Using the wavelength-frequency relationship, we find that the sounds perceived by human ears have wavelength between roughly 17 mm (at 20 kHz) and 17 m (at 20 Hz).

 Standing wave patterns

So far, we have discussed about travelling waves moving from one place to the other. There is, however, a special kind of waves that stand still and only oscillate in amplitude with time, the standing waves. They may be created from two waves (with equal frequency, amplitude and wavelength) travelling in opposite directions. Using superposition, the resultant wave is the sum of the two waves. The movie below shows that the net result alternates between zero and some maximum amplitude. Unlike the travelling waves, the standing waves do not carry energy.

Further wave phenomena

Dispersive waves

Waves can also propagate in bars and plates. In this case, a remarkable fact is all waves do not travel at the same velocity. Higher-frequency waves travel faster than lower-frequency waves. These differences in speed causes spreading or dispersion of wave packets, as shown in the movie below.

Refraction of sound waves (under construction...)

Diffraction of sound waves

The animation below illustrates how a travelling wave emitted from the upper left corner by an airplane at take off is diffracted by a sound barrier erected to shield homes from the traffic noise. This solution is efficient only if the houses are located within the shadow region of the sound barrier.

Radiation from acoustic sources

Monopole sources

As an example of a monopole source, one might think of a balloon that is rhythmically expanding and contracting. The resulting sound field (due to successive compressions and rarefactions of the surrounding fluid) has the same amplitude in all directions. The pressure field produced by a monopole source is shown in the animation below. The air particles move back and forth as the spherical wave expands outwards. In practice, the monopole source model is a good approximation for the sound field  radiated by a boxed loudspeaker at low frequencies.

 Dipole sources

The simplest dipole source consists of two monopole sources of equal strength placed a short distance apart, operating at the same frequency but always 180^o out of phase of each other [*]. An example is given by a bare speaker cone where the volume of air pushed out by the front side is compensated by the new volume of air that is pulled on the back side. The animations below show that a dipole source does not radiate sound equally well in all directions. Sound is cancelled in the regions along the vertical axes.

How is the sound field radiated by a dipole consisting of two monopoles when one of them is delayed with respect to the other by the time it takes for a wave to travel from one monopole to the other ? Answer

The tuning fork

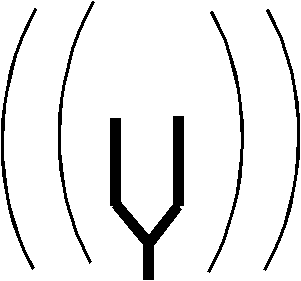
An observer holding and rotating a sounding tuning fork at arm's length from the ear will hear two loud regions in the plane of the tines separated by two quiet regions in the plane perpendicular to the fork. From the animations below, it can be seen that a similar sound field can be produced by a linear quadrupole source. It is made of two opposite phase dipoles lying on the same line [*].

When the observer holds and rotates the sounding tuning fork close to its ear, he then finds four positions where the sound is loud, alternating with four positions where the sound is quiet. The movies below show that a lateral quadrupole source can provide a similar sound field. It is made of four monopoles with alternating phase located at the corners of a square [*].

The baffled piston

An important example is a baffled piston-type source, namely a flat solid disk set in an infinite plane baffle and moving up and down along its axis of symmetry. Analytic expressions can be obtained to describe the sound pressure radiated in the far-field at all frequencies. This model is used for practical baffles such as air boxed loudspeakers.

From the animations below, it can be seen that the radiation patterns of a loudspeaker is different at low, medium and high frequencies.

      - At low frequencies, the sound field radiated  by a loudspeaker spreads out evenly in all  directions.

      - At higher frequencies, the sound pressure produced by a loudspeaker is much contained within a cone of 55^o from the center axes. So, at these frequencies, a listener will perceive higher pressure levels if he is located in front of the speaker. If he moves to either side, he will perceive weaker pressure levels.

       - At much higher frequencies, the sound field radiated by a loudspeaker is constricted within a cone of about 20^o on either side of the central axis. Now, the sound pressure levels fall off very rapidly as a listener steps away from in front of the loudspeaker. In practice, different frequencies are sent by different subwoofers and so, it is unlikely to observe such a difference in sound levels.